Messages

Congratulations Oricorio on your win!  Advancing even to semfinals is no joke - it takes a great amount of skill.  I imagine the playerbase has only improved over the years too, so there's a huge amount of competition.  Be proud of yourself!

im 12

i was told to save love live, the most superior of all games

1. Undertale - 4
2. Legend of Zelda: Ocarina of Time - 10
3. Super Mario Bros. 3 - 9
4. Dig Dug - 6
5. Halo 2 - 3
6. Legend of Zelda: Breath of the Wild - 9
7. Splatoon/Splatoon 2 - 11
8. Ace Attorney Something About Justice - 11
9. Danganronpa - 9
10. Super Mario 3D World - 10
11. Mega Man (the Best One) - 11
12. Super Smash Bros. Brawl - 10
13. Super Smash Bros. Brawl, but Subspace Only - 9
14. Ghost Trick - 13
15. Kirby Super Star - 11
16. Legend of Zelda: Wind Waker - 11
17. Kirby's Return to Dreamland - 10
18. Kirby and the Crystal Shards or Something - 10
19. Mario Kart Double Dash - 11
21. Mario Party 6 - 13
22. Duck Hunt - 8
23. Fallout 3 - 7
24. Pokémon Emerald - 10
25. Pokémon Mystery Dungeon: Explorers of Sky - 10
26. Shovel Knight - 10
27. Warioland 2 - 9
28. Love Live School Idol Festival - 7
29. Rhythm Heaven - 10
30. Super Metroid - 10

hul

also plus a constant

Yes, that's exactly correct, InsigTurtle!

Had a physics test today, was briefly confused by a question asking how much work needed to be done to lift an object from the surface of a planet to a distance infinitely far away.

Since you only started electricity, I'm guessing the question you answered likely related to only gravitational energy.  In which case, the answer would just be GMm/R; G is the gravitational constant, M is the mass of the planet, m is the mass of the object, and R is the radius of the planet.  To find the difference in potential energy between two points (i.e. which for the question will tell us the magnitude of work that must be done to bring something from point A to point B), you have to take some sort of reference point (in this case, I chose the center of the planet), find the distances R_A and R_B of points A and B to the reference point, and then plug into GMm/R_A - GMm/R_B.  For your question, R_A = R = Radius of Planet and R_B = Infinity, so that you get GMm/R - GMm/infinity = GMm/R - 0 = GMm/R

Hope you managed to get it right - I wasn't sure based on the wording of your post, but just to be safe!

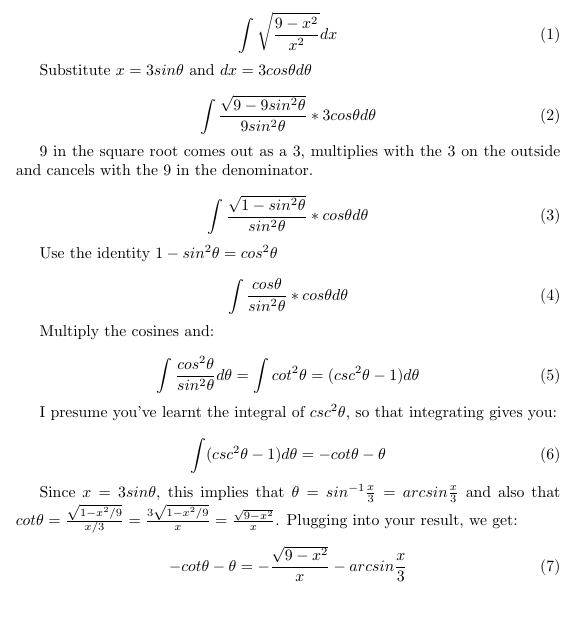
I just want to make sure I'm not doing this completely wrong; we've done a few problems similar to this, but none completely identical:

The problem is "Find dy/dx of ∫ sqrt(t) dt, with upper limit being tan x, and lower limit being 0," and my answer is sec^2 (x) sqrt(tan x).

Your answer is correct.  This is the Fundamental Theorem of Calculus joined with Chain Rule is that helps you understand it better.  An easy way to check would be to evaluate yourself by taking the integral and then differentiating.  However, as a word of fair warning, you might see in the future examples where you have to differentiate a function that doesn't have an elementary integral (e^x^2, as a common example), so it'll be useful for you to understand the general process for finding the solution than doing straight evaluations.

Lol math minor and I haven't seen that ages and I'm too lazy to try. If you could take it now, it's better than taking it later, the high school AP stuff are jokes compared to the college courses.

Anyway, a professor assigned this to her NON-MAJOR chemistry students (CHEM 100) and I'm wondering how they can even do this when I had to look it up myself. I need to ask her to be sure lol, that's how bad it is xD I don't have their book so I've just been using the internet, hope their book actually explains it.



(Name the compound using IUPAC nomenclature)

2-Acetyl, 4-Methyl, Cyclohexene-1-ol???  idk, taking a guess lol

Poll is up.

Presuming NocturneofShadow can balance his game for 8 people, I'll put up the poll tomorrow at 11PM EST.

Hopefully, I don't fall asleep on a subway train on my way back home, haha.

my computer is lagging rip me

Players:
1. BlackDragonSlayer
2. Dudeman
3. E. Gadd Industries
4. AwesomeYears
5. TheMarioPianist
6. BrainyLucario
7. fank009
8. davy

Hosts:
1. NocturneOfShadow

I haven't looked into the game too in-depth, but most everything looks fine to me.

The only thing I would suggest is nerfing the Wolf Painters slightly (presuming you allow them to paint a Player any color) - there's nothing stopping them from just painting themselves Green and making seerings meaningless.  Aside from that, you can feel free to start your game when you wish!

back in my day, we played with as many as -12 people if it meant keeping twg alive!!!  you whippersnappers can't appreciate what u have!

Anyone who would like to host can make a game now - 9 Player game if you're playing, 10 Player game if you aren't.  Ideally, we should start within 48 hours.

hul

1. BlackDragonSlayer
2. Dudeman
3. TheMarioPianist
4. E. Gadd Industries
5. BrainyLucario
6. NocturneOfShadow
7. davy
8. Yellow
9. FireArrow
10. 12Mari0

Yup, sounds good!  Go ahead and make the game! (sun)